Broadband Source Parameters Computed at the NEIC

Since 1985, the NEIC has been actively incorporating methods of broadband seismogram analysis into its routine operations with two objectives: (1) to improve the accuracy of reported source parameters, such as depth and focal mechanism; and (2) to introduce new data services that were previously impractical without digital broadband data, such as estimating radiated energy. Some of the broadband digital operations in routine use by the NEIC are described below.

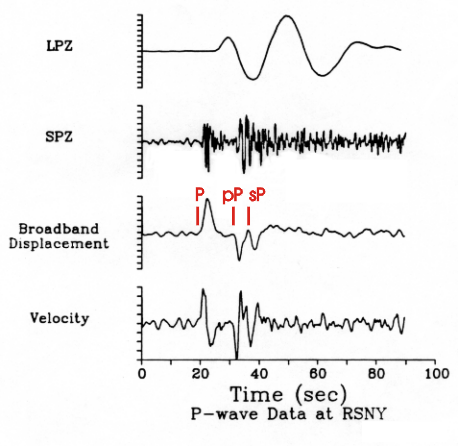
Broadband depths. From digitally recording networks and arrays, broadband seismograms of body waves that are flat to displacement and velocity in at least the frequency range from 0.01 to 5.0 Hz are now routinely obtained for most earthquakes of magnitude greater than about 5.5 by using the method of Harvey and Choy (1982). The broad spectral content in displacement and velocity records often permits identification and resolution of the source functions of direct and surface reflected phases by direct inspection. The advantages that accrue from analyzing such data can be seen in an example (Fig. 1) that compares different representations of the same P wave. The arrival times of direct and surface reflected arrivals are most easily identified and measured in broadband displacement and velocity.The differential times can be used to infer source depth. Polarities and relative amplitudes of various phases can be measured directly from the data and used to constrain nodal planes of the focal mechanism.

Figure 1. Four representations of a P wave recorded at station RSNY are shown. From top to bottom: the long-period record, short-period record, broadband ground displacement and broadband ground velocity. In the broadband records the identification of the depth phases is unequivocal and the measurement of differential travel times with respect to the direct P wave is accurate.

Since October 1985, estimates of depth determined from inversion of differential times of depth phases identified on broadband waveforms for earthquakes having m_b > 5.8 have been published in the Monthly Listings of the Preliminary Determination of Epicenters (PDE). Since January 1996, the broadband depths for shallow earthquakes have been derived by modeling broadband P and transversely polarized S waves using methods described by Choy and Dewey (1985).

Broadband fault-plane solutions and complexity of rupture. Beginning January 1996, a broadband fault plane solution has been determined whenever possible for any earthquake having a magnitude > 5.8 and published in the Monthly Listings of the PDE. The broadband fault-plane solution is determined primarily from least-squares fitting of synthetic waveforms to teleseismically recorded broadband body waves that are flat to displacement between approximately 0.01 to 5.0 Hz. Additional constraints are sometimes provided by polarities from P-waves at distances < 30° from the epicenter, from Hilbert-transformed body waves of certain secondary arrivals (e.g., PP), and from transversely polarized S waves. Prior to January 1996, fault plane solutions were constrained primarily by using first motion data from P, pP and PKP waves. These solutions are "first motion" solutions rather than "broadband" solutions.

Along with the depth and mechanism analyses, the NEIC examines the source-time functions of P-wave displacements for complexity of earthquake rupture. The identification of any multiple events is noted in the COMMENT section of the description of the earthquake in the Monthly Listings.

Radiated Energy ( E_S ). The energy radiated by an earthquake is estimated from the energy spectral density of the broadband P-waves, using the method described by Boatwright and Choy (1986), where the energy flux in the P waves is obtained by direct integration of velocity-squared records.

Energy gives a physically different measure of earthquake size than moment. Energy is derived from high frequencies in the velocity power spectra, while moment is derived from the low-frequency asymptote of the displacement spectra. Thus, energy is a measure of seismic potential for damage, while moment is a measure of the area ruptured by an earthquake.

Energy Magnitude ( M_e ). The energy magnitude, M_e, is computed from the radiated energy using the Choy and Boatwright (1995) formula (eq. 6):

where energy is in Newton-meters.

The relationship of M_e to M_W. Although M_e and M_W are magnitudes that describe the size of an earthquake, they are not equivalent. M_e, being derived from velocity power spectra, is a measure of seismic potential for damage to anthropogenic structures. M_W, being derived from the low-frequency asymptote of displacement spectra, is physically related to the final static displacement of an earthquake. Because they measure different physical properties of an earthquake, there is no a priori reason that they should numerically equal for any given seismic event. The energy magnitude, M_e, is an essential complement to moment magnitude, M_W, for describing the size and affect of an earthquake rather than an alternative.

Starting with the November 1986 Monthly Listing, the NEIC has published estimates of radiated energy computed directly from broadband body waves. M_e has been published routinely beginning with the July 1995 Monthly Listing.

References

Boatwright, J. and G. Choy, Teleseismic estimates of the energy radiated by shallow earthquakes, J. Geophys. Res., 91, 2095-2112, 1986.

Choy, G. L., and J. L. Boatwright, Global patterns of radiated seismic energy and apparent stress, J. Geophys. Res. 100, 18205-18228, 1995.

Choy, G. L., J. L. Boatwright, and Steve Kirby, The radiated seismic energy and apparent stress of interplate and intraplate earthquakes at subduction zone environments: Implications for seismic hazard estimation, U. S. Geol. Survey Open-File Report 01-005, 10 pages, 2001.

Choy, G. L. and J.W. Dewey, Rupture process of an extended earthquake sequence: Teleseismic analysis of the Chilean earthquake of 3 March 1985, J. Geophys. Res.,93, 1103-111, 1988.

Harvey, D. and G. L. Choy, Broadband deconvolution of GDSN data, Geophys. J. R. Astr. Soc., 69, 659-668, 1982.

Current USGS Energy and Broadband Solutions